Hall algebras are Grothendieck groups

I’ve been attending a seminar/class run by Nick Proudfoot preparing for his workshop this summer on canonical bases. In conversations with Nick and graduate students, and there’s been some confusion about the relationship between Hall algebras and Grothendieck groups. Obviously, if you read the definitions you’ll see they are not the same, but the idea seems to be floating around that there is something going on with them. At some point, I decided writing a blog post on the subject would be a good idea. What are Hall algebras?

The Hall algebra of a category is the Grothendieck group of constructible sheaves/perverse sheaves on the moduli stack of objects in the category. The Hall algebra is an algebra because the constructible derived category of the moduli stack of objects in abelian category is monoidal in a canonical way.

To my mind, this is what makes Hall algebras worth studying, yet it’s oddly ignored in the literature on them (as far as I know; people should feel free to correct me). For example, it’s never mentioned in Schiffmann’s Lectures on Hall Algebras, the closest thing the subject has to a standard reference.

A bit more detail

So, let me unpack all of this. What people will usually tell you is that the Hall algebra is the functions on the set of objects of an abelian category. Important examples include the category of representations of a quiver and the category of coherent sheaves on a projective curve. So, when I say it actually has to do with the Grothendieck group of constructible sheaves on the moduli stack of objects, I have to explain two things:

  1. Why are the set of objects in an abelian category actually a stack?
  2. What do functions on a space have to do with the Grothendieck group of sheaves on said space?

Luckily, I’ve already addressed 2. in another blog post. Roughly, given a complex of sheaves with constructible cohomology, you can take the Euler characteristic of the stalk (or much better, the super-trace of Frobenius on the stalk) and get a function, which obviously only depends on your class in the Grothendieck group.

So the question is why the set of objects is secretly a stack; as usual, I’m sure I will get some technicalities wrong if I try to work in full generality, so let me restrict to the question of why a finitely generated algebra A over a field k has a moduli stack of finite dimensional representations. In this case, the space of A-actions on k^n has a very natural structure as an affine variety; pick generators a_i\in A, and create n-by-n matrices \alpha_i full of formal variables for each of them. Now, take the list of relations the a_i satisfy; maybe a_1^2=0. Look at the corresponding product of the \alpha_i‘s (in our example \alpha_1^2) and set the entries of that matrix to 0. That’s some polynomial equations in the entries of the matrix, and they define an affine variety R_A^n whose points over any commutative k-algebra are n-dimensional representations (with fixed basis!) of A over that base.

But, I’ve overcounted! Lots of these are isomorphic representations with different choices of bases. Luckily all isomorphisms come from changing basis on k^n, that is, acting by GL_n(k) on R_A^n by conjugating matrices. So really, I want R_A^n/GL_n(k); but we have to think about what this means. It’s not a variety, sadly that’s not how quotients of varieties by groups work.

Instead, it’s a stack. This is really just a recognition of the fact that objects in categories aren’t just inert things; they have automorphisms (which in this quotient R^n_A/GL_n(k) show up as the stabilizers of points). So this shows, modulo your willingness to accept the function/sheaf correspondence that the Hall algebra is the same thing as the Grothendieck group of sheaves on the moduli space of objects. Except, I haven’t said anything about the multiplication.

The monoidal structure

Where does the multiplication on the Hall algebra come from? Well, from a correspondence which looks like this:

R_A^{d''}/GL_{d''} \times R_A^{d'}/GL_{d'} \leftarrow\mathrm{SES}(d'',d,d')\to R_A^d/GL_d

The middle term \mathrm{SES}(d'',d,d') is the stack of short exact sequences in our category with terms of dimension d'', d=d'+d'' and d'; the maps are “take the outside terms” and “take the middle term.”

The Hall algebra multiplication is “pull back functions from the left, and pushforward to the right.” Of course, some care is necessary to make sure we interpret “pushforward” correctly. This is why people often require “finitary categories” for Hall algebras. Then it’s easy to say what “pushforward” should be; it’s just summing over the (finite) fibers of that map accounting for the that that points in R_A^{d'}/GL_{d'} come with automorphisms. There are also some funny powers of q (see Schiffman) which come from fixing things so that pushforward preserves Verdier duality (they arise directly from the virtual dimension of the components of the stack R_A^{d'}/GL_{d'}).

EDIT: Joel points out that this notation is probably needlessly obscure. The point is that I’m looking at the stack of short exact sequences \mathrm{SES} which has three projection maps p_1,p_2,p_3:\mathrm{SES} \to \mathrm{Ob} to the stack of objects given by taking the sub-, total, or quotient objects. Multiplication in the Hall algebra is essentially (p_2)_*(p_1^*f\cdot p_3^*g) (except with pullback adjusted by some funny constants from the Euler form). All I’m doing above is thinking of the stack of objects in more concrete terms. END EDIT

One of the points I at least tried to emphasize in my earlier posts on this stuff is that the Grothendieck trace formula really says that

The action of correspondences on categories of sheaves by push-pull categorifies their action on functions by push-pull

OK, so that’s not literally what Grothendieck said, but maybe it’s what he should have. What structure does the correspondence

R_A^{d''}/GL_{d''} \times R_A^{d'}/GL_{d'} \leftarrow\mathrm{SES}(d'',d,d')\to R_A^d/GL_d

induce on the level of categories of sheaves? Well, it lets one take sheaves on R_A^{d''}/GL_{d''} and R_A^{d'}/GL_{d'}, take outer tensor, pull and push, and get a sheaf (well, really complex of sheaves) on $R_A^d/GL_d$. It’s a monoidal structure on the derived category of sheaves on the sheav on the moduli space, assuming you think of sheaves on each component of the moduli space living together as a happy family. This structure was (for example) extensively studied by Lusztig, sadly, before the language of monoidal categories had gotten popular (well, actually is it popular yet? Anyways, it’s more popular now than in the 80’s). Anyways, I’ve taken the liberty of calling this the Hall monoidal structure for reasons which I think should be obvious.

So the upshot of all this discussion is:
Theoremesque: The Hall monoidal structure on sheaves on the moduli space of objects in a monoidal category categorifies the Hall algebra of the category. Similarly, all obvious subalgebras of the Hall algebra correspond to subcategories of the monoidal category.

I call this “theoremesque” because for a real theorem, I need to be a lot more careful about hypotheses. This isn’t so much a theorem as a principle.  But it’s a good principle; it leads one to construct canonical bases and categorifications of quantum groups, but that’s a story for another post.

13 thoughts on “Hall algebras are Grothendieck groups”

  1. I agree with you that it is worth pointing out and emphasizing this perspective.

    You can find some of your perspective in Joyce’s work (http://front.math.ucdavis.edu/0503.5029). He defines the Hall algebra as the algebra of constructible functions on moduli stack of objects in an abelian category. If you add your point 2 above, then you get your above bold statement.

    By the way, you can considerably simplify your definition of the monoidal structure. Just use the diagram
    Obj(A) x Obj(A) Obj(A)

    where Obj(A) is the moduli stack of objects and SES(A) is the moduli stack of short exact sequences.

  2. Just a reminder that I blogged about Joyce’s work a couple of years ago.

    Final comment: you misspelled Olivier name. It is Schiffmann.

  5. Hi Ben – That’s a great picture, of course, and thanks for doing such a nice job explaining it, but I think it’s well known to practitioners of the subject. In particular it’s rather explicit in the papers of Schiffmann and Vasserot about the elliptic Hall algebra, Macdonald polynomials etc. (and probably various other papers). Of course the best known case of this is the spherical Hecke category in geometric Langlands in the case of $GL_n$ – this is a monoidal subcategory of the Hall category of the curve. In fact the geometric Langlands program (for $GL_n$) is just the study of the action of the “pointlike” subalgebra of the Hall category of a curve on the “vector-bundle like” submodule (I learned this picture -as did Schiffmann-Vasserot I believe – from Kapranov’s paper Eisenstein Series and Quantum Affine Algebras). Anyway Schiffmann-Vasserot use this perspective to great effect, recovering Macdonald polynomials and their DAHA symmetry from geometric Eisenstein series on elliptic curves and their Hecke symmetry, and in fact use their detailed understanding of the elliptic Hall category to prove important instances of geometric Langlands.

  6. Looking back perhaps I misunderstood your point – you’re not claiming this is a new picture, but that it’s insufficiently well advertised, with which I couldn’t agree more. Sorry! Anyway it’s worth mentioning the beautiful paper by Schiffmann, On the Hall algebra of an elliptic curve II (arXiv:0508553), where this picture is discussed explicitly.

  7. David-

    I certainly agree you that there’s nothing truly new in the post above, but I think there’s an important distinction to be made; “experts in the field” (I think it’s a bit of a stretch to call myself an expert in Hall algebras, but whatever) can read Olivier’s paper and the post above, and say “ah, right, they’re saying the same thing.” Hell, this is really all in Lusztig’s papers from 1990. But neither of those papers contains the word “monoidal”, for example. Of course, if you know what a monoidal category is and read Olivier’s paper, you say “ah, that’s a monoidal category.” But how many people know that Hall algebras are actually about monoidal categories?

  8. Thanks for the nice post, Ben!

    So, here is a question. The Grothendieck group of vector bundles on a space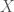 X has a ring structure, where multiplication is given by tensor product. We can extend this to all constructible sheaves if 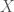X is smooth — although I don’t know what that means in the stack world!

    So now we have two algebra structures: The Hall algebra structure by push-pulling along short exact sequences and the Grothendieck ring structure by tensor product. Do you know if there is a connection?

    I would particularly like to understand this because I would like to understand the relation between http://front.math.ucdavis.edu/0905.0002 and http://front.math.ucdavis.edu/0410.5187 . These are two categorifications of acyclic cluster algebras, where the first uses tensor product and the second uses Hall algebras.

  9. David – that’s a very interesting question! just a couple of simple comments:
    1. There’s no problem defining the tensor product of sheaves on stacks — any construction on sheaves that’s compatible with smooth pullbacks (ie satisfies smooth descent) makes sense for Artin stacks.

    2. The tensor product structure is symmetric monoidal, while the Hall structure is only monoidal in general (though if you take the derived version and your category is Calabi-Yau you have more of a chance to flip around exact triangles), so without some conditions I wouldn’t expect a relation between the two.

    The Hall category has a “convolution” monoidal structure, while your other side has a “pointwise multiplication”, so a relation between them would likely be a kind of Fourier transform. In fact the geometric Langlands correspondence is perhaps a relation of the kind you want. Geometric Satake relates a monoidal subcategory of the Hall category for GL_n with the category of sheaves on pt/GL_n, aka the moduli stack of n-dimensional vector spaces. More generally geometric Langlands (for GL_n over all n) can be thought of as giving a description of the entire Hall category for sheaves on a curve for GL_n in terms of sheaves on the moduli stack of (all rank) local systems on the curve. (To get the full Hall multiplication you have to understand also Langlands functoriality between the different GL_n’s…)

  10. David (Speyer)- The short answer is that I don’t understand what’s really happening in this picture; however, I think there is a lot more to be said here. Another way of thinking about the picture above is to say (roughly) if one category is an additive categorification of something, the Hall category (sheaves on the moduli space of object) of that category should be a multiplicative categorification. Let me make the bold and appropriately vague conjecture that behind any structure seeming to have both additive and multiplicative categorifications is some kind of Hall type picture.

    I know you’ve read that Nakajima paper a lot more carefully than I have, but it seems strange to describe it as “using tensor product”; when described in terms of geometry, doesn’t he say it’s a convolution diagram?

  11. Pingback: Fourth Linkfest ~
  12. Pingback: Distribution, commutation and association in database queries | cartesian product ~
  13. Pingback: Diagrammatic and geometric categorification « Secret Blogging Seminar ~

Comments are closed.