About that field isomorphism…

There was a question about the isomorphism of fields between \mathbb{C} and \bar{\mathbb{Q}}_\ell. I just stuck that in this previous post as a comment, so let me elaborate a tiny bit.

The important thing about this kind of field isomorphism is that it tells you almost nothing interesting. What makes \mathbb{C} interesting is its topology, and when you lose that, \mathbb{C} becomes a rather floppy, uninteresting object.

So, I guess, my point is, this result is helpful as a security blanket, when you have to face the \ell-adics the first time, but it actually has few really important consequences.

Theorem. Any two algebraically closed fields K_1,K_2 of the same transcendence degree \aleph over \mathbb{Q} are isomorphic. In fact, any of isomorphism of subfields (EDIT: of finite transcendence degree over \mathbb{Q}) of K_1 and K_2 can be extended to a global isomorphism. In particular, if a_1\in K_1,a_2\in K_2 have the same minimal polynomial, then there is an isomorphism sending a_1 to a_2.

Corollary. The transcendence degree of both \mathbb{C} and \bar{\mathbb{Q}}_\ell over \mathbb{Q} is the continuum, so they are isomorphic.

I’ll just note, the moral here is that the isomorphism with \mathbb{C} tells you nothing about an element of \bar{\mathbb{Q}}_\ell you couldn’t have found out from its minimal polynomial. I’ll include a proof, just so you see how much non-canonical stuff goes into this isomorphism.

Proof. Pick any isomorphism between subfields of K_1'\cong K_2'. Then pick transcendence bases B_i for K_i over K_i'. Since these have the same cardinality, there is a bijection between them, and thus a field isomorphism K_1'(B_1)\cong K_2'(B_2). Since these are transcendence bases, taking algebraic closure, we obtain an isomorphism K_1\cong K_2.

EDIT: A better proof was suggested in comments, which I have stolen. Thanks, Lior.

10 thoughts on “About that field isomorphism…”

  1. I think you mean to say, “if \aleph is a limit cardinal,” not a successor cardinal. Successor cardinals \aleph are of the form $\latex \aleph’ + 1$; the only cardinals of this form are the nonempty finite ones.

  2. Point of notation: \aleph is the usual notation for the cardinality of the continuum, not for an arbitrary cardinal. If you must, you can use the explicit enumeration \alpha_\alpha for the cardinals, but \kappa is more usual.

    Point of mathematics: if you are willing to induct on the cardinals (and in particular adjoin infinitely many elements at a time) then I don’t see the point of the induction in the first place. Simply take a maximal algebraically independent set on each side. They have the same cardinality. Choose an isomorphism of these sets and extend it to an isomorphism of field extensions. Now use uniqueness of algebraic closures to get all the way to K_i. You can of course take transcendence bases containing your favourite transcendentals a_i.

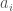  3. Not that this matters at all for your main point, but you also want to make sure you’ve picked $K’_1$ and $K’_2$ carefully enough that the transcendence degree of $K_1$ over $K’_1$ equals that of $K_2$ over $K_2’$. And you could choose $K’_1$ and $K’_2$ poorly, since algebraically closed fields with infinite transcendence degree are always isomorphic to proper subfields of themselves.

    This argument has nothing to do with characteristic zero, the same proof shows that any two algebraically closed fields of the same positive characteristic and the same transcendence degree are isomorphic. It’s also the same proof (morally speaking) as the uniqueness of vector spaces of a given dimension over a given scalar field.

  4. As John Goodrick has pointed out, the sentence in the theorem beginning with “In fact” is false. It would imply, for instance, that C is not isomorphic to any of its proper subfields, but it is easy to to see that this is not the case: take a transcendence base B = {b_i} for C over Q, omit any one element from B to get an algebraically independent set B’ of continuum cardinality, and then take the algebraic closure of Q(B’): this is again an algebraically closed field of continuum transcendence degree over Q, so is isomorphic to C.

    As an aside, it is true that C and the (Q_p)-bar are isomorphic as fields equipped with a Borel structure, i.e., the sigma algebra of sets generated by their topology. I seem to recall that this can be useful, but I forget the specifics.

  6. I don’t like having to choose an isomorphism between (Q_\ell)-bar and C: this always seemed rather violent to me. But then last month I was writing some things up and, sadly, I found I _had_ to choose an isomorphism in order to state everything properly. The issue comes down to the fact that C has a distinguished square root of any positive integer p, but Q_\ell-bar does not.

    The context here is the local Langlands conjectures (LLC) for GL_n, which puts into bijection objects in two rather different-seeming categories: One, representations of the group GL_n(Q_p) of a certain sort, and two, continuous n-dimensional representations of the absolute Galois group of Q_p. I didn’t say what field the coefficients of these representations are in: certainly on the Galois side, it is more convenient to have the coefficients be in an algebraically closed ell-adic field, because that sort of topology is more conducive to rich Galois representations. (If you used C coefficients, the image of Galois would have to be finite!) As for the GL_n(Q_p) side, the notion of “admissible representation” allows you to work over any alg. closed field of characteristic 0. So why leave the ell-adic field? (That’s where exciting etale cohomology has its coefficients, and I want to understand the LLC through that prism anyway.) Who needs C at all?

    I do, apparently. The right way to phrase the LLC is via the formalism of L- and epsilon-factors, which are quantities associated to objects of both categories that must be preserved in the bijection. On the side of representations of GL_n(Q_p), in order to construct epsilon-factors, you need to start with an additive character psi of Q_p, extend this via the trace to the matrix algebra M_n(Q_p), and then find a Haar measure dx on M_n(Q_p) which is self-dual to psi. (Self-dual means: Fourier transform applied twice has to act the standard way.) Uh-oh: if dx is self-dual, then so is -dx! If we’re working over a field other than C, then we no longer have a notion of “positive”! (If you start with any dx and adjust it to make it self-dual, you’ll find you have to take a square root.) So over Q_\ell-bar, the epsilon factors are only defined up to sign. Very sad. Anyone have a fix? Until then, it’s the “nuclear option”: Choose an isomorphism between C and Q_\ell-bar….

  7. Dear Jared,

    You are using the “unitary” normalization of LLC, which
    is *not* invariant under automorphisms of the coefficient
    field.

    There are other choices, which are better suited to comparing
    with l-adic Galois representations. In particular, when one
    looks at the version of LLC that is actually realized in the
    cohomology of Shimura varieties (e.g. in the work of Deligne-Langlands-Carayol for (Hilbert) modular forms),
    it is not the unitary normalization that one finds, but
    one of these other normalizations. (*Which* other one
    depends on your conventions for canonical models
    for modular curves or Shimura curves.)

    I would be happy to discuss this more via email if you
    want more details.

    Cheers,

    Matthew

  8. I would be happy to discuss this more via email if you
    want more details.

    Though you shouldn’t feel as though you can’t discuss it here. The more actual math that goes on here, the better, as far as I’m concerned.

  9. Matthew: Thanks for writing! I’m aware of this distinction between normalizations of LLC. I was wondering aloud if one could construct the theory of epsilon factors on the automorphic side (for representations of GL_n(Q_p), say) in a way that is compatible with automorphisms of the coefficient field. (It is cheating to use C in any way.) On the Galois side, there is no problem: one defines eps(theta, psi, dx) for each F/Q_p, each additive character psi and each measure dx on F, and then extends this definition to all representations using inductivity in degree zero and Brauer’s Theorem. (If anyone reading this has not read Tate’s “Number Theoretic Background” paper … well, put it on your list, I suppose.)

    But I am lost as to how to proceed on the automorphic side without using the crutch of self-dual measures. One could define a quantity eps(pi, psi, dx) for each representation pi of GL_n(F), each additive character psi of F, and each measure dx on M_n(F), but then I wouldn’t know how to phrase the LLC in terms of these.

    As you note, a lot of this has to do with the cohomology of Shimura varieties. That’s how I found myself in this mess, actually. But that’s for another long, tangential comment….

  10. Points of notation in response to Alon Levy and Lior:

    Assuming the Axiom of Choice, the cardinals are well-ordered, and therefore can be indexed by the ordinals. The canonical notation for them is thus to call them \aleph_\alpha, where \aleph_0 is the least infinite cardinal, and the enumeration continues in the obvious way.

    It’s standard to call an infinite cardinal \kappa a successor cardinal iff its index in \aleph notation is a successor ordinal, and a limit cardinal otherwise. Alon Levy is right that the only cardinals that are successor ordinals are finite ones, but there are plenty of cardinals that are limit ordinals but successor cardinals.

    Because of the independence of the continuum hypothesis from every standard set of axioms, and the lack of a consensus on a resolution of it, there is no standard notation for the cardinality of the continuum in terms of alephs other than as 2^{\aleph_0}. However, it’s standard (though much less common) to use beth to define a sequence of cardinals given by exponentiation, with \beth_0=\aleph_0 and \beth_{\alpha+1}=2^{\beth_\alpha}, with limits defined in the standard way. Thus, the continuum can be called \beth_1, though in practice I don’t see this notation a whole lot.

Comments are closed.