This is a page where anyone can suggest topics on which they would like to see us (and perhaps others) blog. We make no promises about following through on suggestions, but will make a good faith effort.
-
Subscribe
Subscribed
Already have a WordPress.com account? Log in now.
How about a discussion of long-distance collaboration tools and methods, beyond just using email and talking on the phone? It seems like there are a lot things that might work, e.g. pointing a cheap webcam at piece of paper, using collaborative text editors (e.g. SubEthaEdit), IM’ing (some clients have LaTeX support, I tnink), virtual whiteboards (e.g. Scriblink.com), but which might also turn out to be useless in practice for all sorts of annoying technical reasons. So it would be interesting to hear from people who have had success or failure with various methods.
[Ed. – answered here.]
As for IMing, both Pidgin (formerly GAIM) and Miranda have LaTeX support by a plugin that takes $$ .. $$-sweeps and dumps them through a LaTeX installation before including them as images.
That said, the request is hereby seconded.
Could you please discuss some of the most important and fundamental theorems in mathematics, and explain why they are important. For example, suppose that you were limited to knowing 5 theorems. Which 5 would give you best insight into the true nature of mathematics?
Maybe you could explain a bit about elliptic cohomology and topological modular forms. Jacob Lurie gave a talk about this at our university a few weeks ago and it seemed like a subject with many interesting things still to be discovered. In particular I’m wondering about promising candidates for spectra representing elliptic cohomology or tmf.
I would be curious about learning more on:
“… many constructions of classical algebra (eg, the theory of modular forms) are beginning to be seen to have deep homotopy-theoretic foundations.”
Click to access Morava.pdf
And then I found a remark about “Mixed Hodge Modules” as char. 0 analogs to “perverse mixed complexes” in char. p. What’s all that? Exists there something analog to Deligne’s “yoga of weights”?
Can you talk little bit about the ISI Journals which reply faster than others, in other words they take minimal time for their decision about acceptance or rejection.
[Ed.- answered here.]
Well, this one is a bit simpler than the others above, but I’d like to see a blog the construction of covering spaces. Everyone (?) knows that nice spaces have universal covers, and we all (?) know the theorems about constructing them. But it’d be nice to see some examples explicitly shown. And, of course, once you’ve shown how to build the universal one, all the others follow by modding out by an appropriate subgroup.
Someone has posted a proof of Riemann Hypothesis on arxiv: http://arxiv.org/abs/0807.0090
Does anyone know this guy? It seems that he is from Brigham Young in Utah.
H,
That Riemann hypothesis paper looks like honest work, rather than just crackpottery, so I’m sure the experts are having a look at it. But I’m not going to get too excited about the proof until a few pros tell me it’s legit.
Can someone explain about category O? Ben, I’m looking at you. I missed your series of talks on it when you were at Berkeley and I don’t really know a lot aboutit. What is it and why should I care?
SGA 7.1, exp. IX, contains (in it’s introduction and section 13.4) remarks about ideas and conjectures of Deligne on a “theorie de Neron pour motifs de poids quelconque”. It would be great if someone would explain that.
Could someone tell me who got the prizes at the European Congress of Mathematics today? And possibly describe their achievements at the level of beginning grad student and/or point to relevant papers? Thanks!
Thomas- That’s a pretty tall order. I got about as far was translating the title (roughly, “Neron theory for motives of any weight”). I think you’re probably better off asking an actual algebraic geometer than any of us.
By the way, the portion of SGA Thomas is refering to can be found here: http://modular.math.washington.edu/sga/sga/7-1/7-1t_313.html
For the person who asked about the ECM, the prize booklet is now online at http://www.5ecm.nl/prizewinnersbook.pdf and contains short summaries of their work and their lecture abstracts. Briefly, the winners are: Avila, Borodin, Green, Holtz, Klartag, Kuznetzov, Naor, Saint-Raymond, Smoktunowicz, and Villani.
In case someone knows a good japanese encyclopedia of mathematics (in japanese, for learning reading japanese math), I’d be happy about the bibliographic infos, incl. the ISBN, so that I can ask the library here to obtain it.
L Theory (K theory of quadratic forms). I’ve never understood it (despite considerable effort at one point), and I want to understand it on a conceptual level, and what it’s good for!
Dears, ” to the html board of my blog, I really cannot see what he produced…Can you help me? Here is my blog(http://ugeesana.wordpress.com/), thank you.
” to the html board of my blog, I really cannot see what he produced…Can you help me? Here is my blog(http://ugeesana.wordpress.com/), thank you.
Can anybody told me how to type latex on the blog? I saw the announcement here(http://faq.wordpress.com/2007/02/18/can-i-put-math-or-equations-in-my-posts/), but when I copy the code”
….but I can see the result here>< why?!
ugeesana: WordPress’ FAQ has an entry about this.
I just put a post with LaTeX in a comment thread on your blog and it seemed to work fine. Does this not work for you?
(A few points: I find wordpress’s “Visual” editing environment works better than the “HTML” environment. It might not work to copy the HTML source from our blog to yours, because the HTML works by linking to images on the wordpress server and I’m not sure that you have access to them. However, you can see our latex source by holding your mouse over one of our equations, in which case you can copy that LaTeX source.)
Hi,
Maybe you could tell something about current research programs? That might be interesting, I haven’t even seen a nice list of them yet.
Anton,
Could you make your question more specific? For example, did you mean large-scale long-term structures like the Langlands program, or our personal projects (or those of people we know)?
A good sample of fashionable problems can often be found by looking at the websites of institutes like Fields, IAS, or MSRI, since they tend to bring in specialists for 6 months to a year at a time for special programs. AIM often puts open problem lists on their workshop pages.
Scott,
Sure, I’m talking about large-scale. Langlands, Mirror Symmetry and others. Thank’s for the links, some of them I didin’t know before.
Dear Speyer,
Thank you for coming here to test and tell me. Gee can use latex in my post after try it now
Could someone explain root systems or quivers or like all of representation theory?
I could, but then I would have to kill you….
But seriously, a little bit about quivers I may be able to handle. If I ever finish all the papers I’m working on.
My reply is similar to the second part of Ben’s :).
I don’t think I’ll be writing any blog posts for a while, but I’ll move quivers to the front of the line for when I do. In the mean time, here are two good references: Derksen and Weyman’s article in Notices and Kac’s paper “Infinite root systems, representations of graphs and invariant theory” in the 1982 Journal of Algebra (does not seem to be available online).
Ok David. I think I found a copy http://gdz.sub.uni-goettingen.de/no_cache/dms/load/img/?IDDOC=171997
Hi Guys,
This problem/question interests me for a long time.
Do all primes divides a Mersenne number?
All composites of course is multiple of a prime, but a group or set of composites say like sums of squares, Fermat numbers or function of composite would have a subset of primes as factors.
E.g. 7 does not divide any Fermat number or sums of squares, it seems.
I would love to see your opinion on this.
Regards
Jaime,
I think you are asking whether every prime number divides a number of the form
divides a number of the form  for some
for some 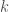 . If so, the answer is yes (except for
. If so, the answer is yes (except for 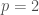 , of course). By Fermat’s Little Theorem,
, of course). By Fermat’s Little Theorem,  divides
divides  .
.
If you meant to require that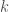 be prime as well, the answer is no. It is easy to check that
be prime as well, the answer is no. It is easy to check that  divides
divides  if and only if
if and only if  divides
divides 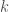 . Since no prime is divisible by
. Since no prime is divisible by  , there is no prime
, there is no prime 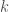 such that
such that  divides
divides  .
.
Regarding sums of squares, you are indeed correct that does not divide
does not divide  unless
unless  divides both
divides both  and
and 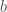 . Let me encourage you to try factoring a lot of numbers of the form
. Let me encourage you to try factoring a lot of numbers of the form  ; there is a very nice pattern in which primes show up. I’ll tell you what it is if you like, but it might be more fun for you to find it yourself.
; there is a very nice pattern in which primes show up. I’ll tell you what it is if you like, but it might be more fun for you to find it yourself.
The discussion of quivers is posted.
Dear David,
Thank you for the elegantly simple proof. Somehow I knew about the connection with the Fermat’s Little Theorem but didn’t see the way you did it.
I think there is a big consequence on this though. If all primes divides all composites and Mersenne primes divides itself. Then there is a direct link to all primes to all Mersenne numbers. Then I think the distribution of primes is linked to the distribution of Mersennes.
And the number of primes is equal to the number of mersennes. On the lower range of course there are more primes because Mersenne with prime order some are composite, but as the primes becomes scarce there numbers almost equal.
This is a bold statement and I would like to hear from you again.
Again thank you.
Best
What is a moduli space?
I assume you have already read David Ben-Zvi’s excellent article?
Yes, in fact I have. I thought it would be a good topic for your blog, but I see your point that an accessible treatment of the subject is already available.
What is a moduli stack? What is a moduli n-stack? Which moduli stacks are algebraic? How does one determine this in general? How does one carry out the general approach in some important special cases? What are the techniques for studying moduli spaces which are special to characteristic 0? What techniques are special to positive characteristic? What is the historical background of moduli spaces (in particular, where did the term “moduli” come from)? What is the current status of moduli of higher-dimensional varieties? What are the basic open problems for moduli of higher-dimensional varieties?
At first I thought this was a joke or some kind of brain dump, but now I think you are making the point that David Ben-Zvi’s article doesn’t cover a lot of questions that you (and other mathematicians) find interesting or even central to the study of moduli. I’m afraid most of them are “above my pay grade” and in particular, the notion of n-stack is, as far as I know, not precisely defined. We can safely say that a moduli n-stack is something that parametrizes families of geometric objects of an (n-1)-categorical nature, but I don’t really know what that means, even when n=1.
Well, I certainly don’t think all of Jason’s questions could be answered in one blog post, and I don’t know enough to answer any of them right now. But they are the sort of questions that I was (badly) hinting at with my question to Nick: what sort of things do people want to know about that aren’t in David Ben-Zvi’s great intro?
Here are some blog posts that I could imagine writing or, even better, could imagine some of the rest of you writing:
* What are the differences between a fine moduli space, moduli stack, coarse moduli space and a miniversal deformation space? What theorems exist relating them? I basically know this stuff, though I’d want to look up a lot of technical points.
* What are some specific, well worked, examples of moduli spaces of higher dimensional varieties? (Polarized abelian varieties, hyperplane arrangements, curves in the plane.) Why is, for example, the moduli space of polarized K3’s considered so much harder to work with? I only have a vague knowledge of this stuff, but I’ve been wanting to learn. I wonder if Paul Hacking could be convinced to write a guest post…
* There has been a great deal of progress on the minimal model program recently. What does it imply for moduli spaces of higher dimensional varieties? I don’t know this stuff at all, but I bet it would make a good blog post if some one else wrote it.
As for Jason’s questions about characteristic dependence and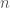 -categories, I’m afraid I don’t even know enough to know whether they could be answered in blog posts. But maybe some of you do?
-categories, I’m afraid I don’t even know enough to know whether they could be answered in blog posts. But maybe some of you do?
There is the Jardine-Toen approach to $\infty$-stacks, which defines the category of oo-stacks as the localization of the category of simplicial presheaves on stalkwise weak equivalences of simplicial sets.
Then of course there is Lurie’s “Higher topos theory”, which really is meant as “Higher Grothendieck-topos theory” i.e. higher sheaf theory. I suppose that solves the question of what an (oo,1)-stack is.
Building on Ross Street’s Categorical and combinatorial aspects of descent theory one obtains a nicely elegant and concrete formulation of the descent condition for “rectified omega-prestacks”, i.e. for presheaves with values in strict oo-categories. That leads to a notion of omega-stacks then. While not being fully general, one can do nice things with that. I am currently using this for talking about differential nonabelian cohomology.
Our hope is that, using Simpson’s conjecture and the fact that every oo-stack should be equivalent to a rectified one (where the restriction maps associate strictly) all one needs to do about omega-stacks in order to get full general oo-stacks is to allow them to take values in omega-categories with weak units.
Dear all,
I wasn’t making a joke or anything. Those are the questions I would have liked to hear the answers to (even if I didn’t know the precise meaning of the question) when I was learning about moduli. There are excellent references out there. But there are few that really explain the key techniques and illustrate them thoroughly on important special cases.
On a more mundane note – the conference list is a bit outdated. Do you guys know of anything exciting happening next spring or summer?
Urs,
Is there are good reason why every infinity stack should be equivalent to a rectified one? I know very little about higher categories, but I was under the impression that strictification is a bad idea or prone to failure beyond 2-categorical constructions.
I recently learned that Clark Barwick has a “well-behaved” theory of (infinity,n)-categories based on a generalization of complete Segal spaces, and some details are written up in the beginning of Jacob‘s recent paper: (infinity,2)-categories and Goodwillie calculus. I don’t know how this precisely relates to moduli stacks, since questions of geometries and topoi with non-invertible higher morphisms are quite beyond me.
Yes, I think one expects a higher dimensional Yoneda lemma. For X an n-stack its rectification should be Hom_{nStacks}( Repr(–), X ), where Repr is supposed to denote the functor embedding the underlying site into n-stack over it.
I wouldn’t put it as general as that. More concretely, for stacks one should, I think, beware that among the two characteristics: they are preshaves with values in categories
a) they satisfy higher descent
b) they may respect compositiion possibly only weakly.
the first one is the essential one capturing the point of stacks as higher sheaves, while the second one is there more for technical reasons.
Compare for instance the Jardine-Toen approach to infinity-stacks which I mentioned: this is based on presheaves with values in simplicial sets. The simplicial sets represent the oo-categorical values (afte localization) but the presheaves themselves are true presheaves, i.e. true functors, i.e. strictly associative. Still, these gadgets can model oo-stacks in this setup.
Hi,
I have been struggling to understand the uses of special functions their representation theoretic significance and geometric significance. I haven’t really succeeded in finding a clear overall view. More significantly I couldn’t find any good reference that gives a unified survey.
I will be really benefit from such an exposition.
Thanks
I would like to hear about Toric Varieties and their relationship to Amoebas (or the other way around). For context, I am aiming at reading the papers on Tropical Geometry by Gregory Mikhalkin and others. Another word I get stuck on is “Gromov-Witten Invariant”. I have probably said a mouthful.
You can understand a lot about amoebas (and tropical geometry) without knowing anything about toric varieties. Most of the time, all you need to know is the connection between the Newton polytope of a polynomial and its amoeba or tropicalization.
Results about polynomials with given Newton polytope can be rephrased in terms of sections of line bundles on toric varieties, but that formulation isn’t helpful in the early stages.
I can see two posts to write here:
(1) My standard intro to toric varieties. I’ve taught people the toric formalism a bunch of times now. I should definitely do a blog post on this.
(2) An intro to amoebas and tropical hypersurfaces, developing the relevant technology in parallel. I can also definitely do this.
As always, don’t hold your breath :).
Some grad — I’m not sure what you’re looking for. My best guess is that you want to understand how modular/automorphic forms show up in representation theory. I’m not qualified to write that, but Scott Carnahan is. Is that what you are looking for? If you don’t know exactly what you are looking for, can you start by telling us what sort of special functions you care about?
Hi John,
I’ve been meaning to write about Gromov-Witten theory for a while now. I’ll put together a post sometime over the weekend.
Though it’s stalled due to some administrative stuff, I’m writing up my lecture notes that (with a few gaps) prove Kontsevich’s formula and posting them. They’ll probably all be scheduled for posting, at least, over the course of next week. All will be up before New Year’s.
Hi David,
First I apologize for a dumb mistake. I meant symmetric functions and not special functions.
Having said that I wanted to understand how they arise in various geometric situations and the interplay between their combinatorial properties and the geometry of Grassmanian.
On the representation theory side I looked into the monograph “Symmetric functions and orthogonal polynomials ” but guess I got lost in the myriad of combinatorial identities.
To put it in a concise way I am probably seeking a motivation/exposition about the connection between combinatorial properties of the symmetric functions and how they determine and are determined by the geometry of flag varieties and the related representation theory of Gl_n.
Thanks
No need to apologize, just trying to understand!
Here is a quick answer, and some references. Note that I am being sloppy about boundary cases, and about what category I am working in; I think it is probably better to give the big picture first and then get the boundary cases right.
So the question is to explain why the following objects match up:
(1) Symmetric functions in d variables.
(2) Representations of GL_d.
(3) Vector bundles on G(d, infinity).
(4) Cohomology clases on G(d, infinity).
More precisely, we have four rings, each of which has a natural sub-semiring.
(1) Symmetric polynomials in d variables. The sub-semiring is symmetric functions which are positive in the Schur basis.
(2) The semiring of representations of , with direct sum as addition and tensor product as multiplication. We get the ring by adding formal negatives of repreentations.
, with direct sum as addition and tensor product as multiplication. We get the ring by adding formal negatives of repreentations.
(3) The semiring of complex vector bundles on . Again, direct sum is addition, tensor product is multiplication and we get the ring by adding formal negatives. This is called K-theory.
. Again, direct sum is addition, tensor product is multiplication and we get the ring by adding formal negatives. This is called K-theory.
(4) The cohomology ring of . Here the sub-semiring is cohomology classes of analytic (or algebraic) subvarieties.
. Here the sub-semiring is cohomology classes of analytic (or algebraic) subvarieties.
The four rings are basically isomorphic. (Basically? Remember what I said about sweeping some issues on the rug. But if I did everything right, then they would be isomoprhic.) As additive semigroups, each of the semirings is . Thus, they have canonical additive bases, and the isomorphisms must take one base to another.
. Thus, they have canonical additive bases, and the isomorphisms must take one base to another.
I wrote about the connection between (1) and (2) here. That post also talks about the relation to flag varieties.
The relation between (2) and (3): The relation is that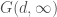 is
is  . Here
. Here 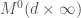 is the space of
is the space of  matrices of rank
matrices of rank 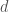 . Vector bundles on
. Vector bundles on 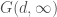 correspond to vector bundles on
correspond to vector bundles on 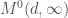 equipped with a
equipped with a  action. Now,
action. Now, 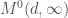 turns out to be contractible. If you know enough topology, this gives you an equivalence between vector bundles on
turns out to be contractible. If you know enough topology, this gives you an equivalence between vector bundles on 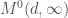 with a
with a  action and vector bundles on a point with a
action and vector bundles on a point with a  action. A “vector bundle on a point” is just a vector space, so this is a fancy way of talking about ring (2).
action. A “vector bundle on a point” is just a vector space, so this is a fancy way of talking about ring (2).
Connecting (4) to the other three is much harder. A sign of this is that the connections between the other three generalize to all reductive groups, but the connection to (4) never do. I should say that, for any space X, there is a relation between the ring of vector bundles on and the cohomology ring of X, called chern character. But this is not the isomorphism I want; it does not play correctly with the semi-ring structure. There are a number of papers which try to give the simplest explanation of why (4) matches the others, my favorite is by Harry Tamvakis.
Dear Hosts and readers;
Pretty sorry to bother you.
I just posted a question (response 47)
on AJ’s article on stacks (2008/06/19)
https://sbseminar.wordpress.com/2008/06/19/whats-a-stack/#comment-4336
Any illumination or reference guide is appreciated.
Best thanks.
— Sincerely, CHL, 2008.12.06
Thank you David for the references.
I will also mention a curiosity that I have had for sometime now. It may sound stupid but let me try and express it anyway (after reading AJ’s post on GW invariant’s).
What is a Field theory ( assuming it is more basic than QFT ,CFT and TQFT) and why mathematicians should at all care about it? Now I am not asking for a formal definition what I want to understand is that what is the motivation behind defining these objects and what is their mathematical significance.
Could someone explain (in terms that an algebraic geometer could understand) how the theory of quivers is used to classify indecomposable torsion-free modules over the completed local ring of a simple curve singularity
(as is done in Green and Reiner’s paper _Integral Representations and Diagrams_, Jacobinski’s paper _Sur les ordres commutatifs avec un nombre fini de resaux indecomposables) or Greuel and Knoerrer’ paper _Einfache Kurvensingularitaeten und torsionsfreie Moduln_).
Thanks!
Some Grad wrote:
Once upon a time, a “field” was a function from a manifold called “spacetime” to some vector space. The classic example is the electromagnetic field. A “field theory” is a bunch of equations satisfied by some field. The classic example is Maxwell’s equations, which the electromagnetic field satisies.
Subsequently, this simple idea of field theory has been generalized and extended in many directions. The most important and shocking generalization is “quantum field theory”. The old idea of field theory described above is now called “classical field theory”. Quantum field theory is similar, but mind-bendingly different.
First of all, everything in the universe is made of fields. So, you are made of fields. You should want to understand yourself. So, you should want to learn field theory.
Second of all, even if you don’t want to understand yourself or the universe, the attempt to understand field theory has led to astounding developments in mathematics ever since field theory was invented sometime in the 1700s. Differential equations, Hilbert spaces, Riemannian geometry, connections on vector bundles, operator algebras… none of these subjects would have developed to their current glory without field theory. Trying to understand these subjects without knowing some field theory is like trying to learn musical scales without ever listening to music.
So, you need to learn field theory.
Dear Professor Baez,
What a beautiful answer that was. I am a math grad student looking to learn some field theory. What math books would you recommend. Please also let me know the math background required. I know no physics beyond high school AP physics.
Thank you for the beautiful answer. The last line about music really touched me.
Hi,
Can anyone give an idea on this equation that relates to Fermat’s Last theorem diophantine equation: a^n + b^n = c^n, where a,b,c,n all positive integers and n > 2. The equation being,
gcd(c^n – 1, a^n + b^n – 1) =1.
Can anyone factors out or show a counterexample that is greater than 1 that is positive ODD integer?
Thanks
Jaime,
The “requests” page is intended for suggested blog topics, although we are open to the possibility of some peripheral discussion. If you have specific questions in number theory, the NMBRTHRY list (find it on Google) would be a better home. However, it would be best if you took some time to make your question more precise. For example, in the above question, you did not specify which number you wanted to be odd, or what form a counterexample should take.
Hi Scott,
Thanks for suggestion I’ll look into it. Regarding the question above I mean the gcd equation implies the FLT, more specifically if a greater than 1 positive Odd factor is common with both functions, it seems will imply that FLT is not true. The form of counterexample is simple positive ODD integer. This second equation is based on Fermat’s Little Theorem. I am wondering whether these functions are known, the first function, c^n – 1 is similar to cyclotomic binomials and I’ve seen this before but not the a^n + b^n – 1 function. Have you seen the second expression before?
It seems that the gcd is always 1, the proof is beyond me although I’m am working on ‘grammar’ model (combinatronics aspect) and too early for me to say.
Again thanks.
I’m still not sure if I understand you correctly, but the following is made out of all odd integers: gcd(7^3-1, 5^3+5^3-1) = 3. Other examples are quite easy to compute, and they don’t say anything about the validity of Fermat’s Last Theorem or Fermat’s Little Theorem (both of which are true).
The equation a^n + b^n – 1 = 0 has rational solutions that give solutions to the Fermat equation (i.e., the only ones are trivial), but plugging integers into the variables and taking the gcd with integers of the form c^n-1 seems to be a rather meaningless exercise.
A general principle is that if you want to solve an “interesting” diophantine equation, then elementary tricks like taking gcds or using congruences will not work, because of the possibility of local-global obstructions. For example, Selmer showed that the equation 3a^3 + 4b^3 + 5c^3 = 0 has solutions mod N for all positive integers N, but no integer solution.
Could someone talk about Schubert Calculus? It sounds rather musical, but really it has to do with Grassmanians. Also, why might someone want to integrate with respect to Euler characteristic?
Can any of the geometric Langlands aficionados give a glimpse of how some of the geometric intuition is used in the original number theory setting?
Most of these questions came from reading Frenkel’s review hep-th/0512172
Grothendieck’s fonctions-faisceaux [function-sheaf] dictionary:
Given a complex of Weil sheaves on a variety over a finite field we can get a function by taking trace of the frobenius element applied to the complex.
How do we go the other way? Are all of these sheaves sums of sky-scraper sheaves and their translates?
How is Deligne’s l-adic Fourier transform related to the Fourier-Mukai transform? Are their any easier applications of Deligne’s FT than the Weil conjecture for curves?
References that should help, but are impenetrable to me:
[1] Kiehl and Weissauer’s book on etale cohomology
[2] Katz: Travaux de Laumon
[3] Laumon: Transformation de Fourier, constantes d’equations fonctionnelles et conjecture de Weil
Thanks!
Richard-
I’ll try to write a more serious answer to these questions soon, since it’s something I was already thinking about. Just to quickly answer your questions:
Given a complex of Weil sheaves on a variety over a finite field we can get a function by taking trace of the frobenius element applied to the complex.
This is true, though you might want to think about it slightly differently; if you have a variety defined over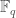 , and a Weil sheaf on it, you want to think about not just the
, and a Weil sheaf on it, you want to think about not just the 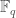 -points, but the
-points, but the 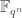 for all $n$. These are the fixed points of the $n$th power of the Frobenius of the
for all $n$. These are the fixed points of the $n$th power of the Frobenius of the  -points of the variety, so you can take the trace of Frobenius on the stalks at those points to get a function on these for all $n$. You want to think about all these simultaneously.
-points of the variety, so you can take the trace of Frobenius on the stalks at those points to get a function on these for all $n$. You want to think about all these simultaneously.
This is a bit like how knowing the traces of powers of a matrix tells you all its eigenvalues, whereas just knowing the trace of the matrix itself tells you a lot less.
How do we go the other way? Are all of these sheaves sums of sky-scraper sheaves and their translates?
So, I think from the above, you can see that this is not going to get you everything you want. You can arrange skyscraper sheaves to work for a few values of $n$, but you can’t get all of them simultaneously. I’m not sure exactly how to describe the image, but it’s a bit trickier than what you said.
How is Deligne’s l-adic Fourier transform related to the Fourier-Mukai transform?
I’m not sure there’s any connection other than analogy. They even generalize different versions of Fourier transform (Mukai is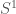 Fourier transform, and Deligne is
Fourier transform, and Deligne is  Fourier transform).
Fourier transform).
Are their any easier applications of Deligne’s FT than the Weil conjecture for curves?
I don’t know about easier…Deligne’s theory of weights is a lot cleaner on projective varieties with no odd cohomology, but on the other hand that opens a whole higher dimensional/intersection cohomology can of worms that may not interest you.
By the way, you may want to look at the “function-sheaf correspondence” section in my recent paper with G. WIlliamson. It’s concise, but at least it’s shorter than Kiehl and Weissauer.
I was wondering if anyone would give an exposition of canonical bases in quantum universal enveloping algebras, plus some/any applications? My personal interests would enjoy applications to integrality/calculability of knot invariants etc. , but anything would be great.
Thanks a lot for the great blog!
Does anyone happen to know how to write down a point in the affine Grassmannian? More precisely, I’m looking for an analog of the canonical form for kxn matrices in the Grassmannian setting, but I am at a complete loss as to how to do this in the affine case. A post about how to think of points, lines, etc in the affine case would be fantastic. Thanks!
As a sporadic blogger (under a mild pseudonym), I know how nice it is to receive a comment — even one (such as this) with no added content. So, I will relate how impressed I was reading this blog for the first time. Of course I don’t know much about the subject(s), but the blog is exactly what a bunch of really smart math friends can do with organizational and pedagogical talent.
Hmm… trying to say something math-y now…. Nope, got nothing. Just a request — opers, what and why in under 100 words.
Thanks!
why do people care about sheaves? and how are they perverse? also what is a scheme.
mohammed akbari sehat writes:
I think that Selig’s notes do a good job walking you through the basic definitions quickly. If you want to get some motivation for the definition, you might like Scott’s old post Group = Hopf Algebra.
Do you know the motivation for the definition of a Hopf algebra, by thinking about functions on abelian groups? If is the vector space of
is the vector space of 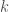 -valued functions on a finite abelian group
-valued functions on a finite abelian group 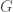 , then we have the following operations: inclusion
, then we have the following operations: inclusion on every element of
on every element of 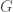 , giving a map
, giving a map 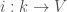 ; pointwise multiplication of functions, giving a map
; pointwise multiplication of functions, giving a map  ; evaluation at the identity, giving a map
; evaluation at the identity, giving a map  ; the map
; the map  , giving a map
, giving a map  ; and the map
; and the map  , giving a map
, giving a map 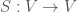 .
.
of the function which is
If you think through what properties these maps obey, you’ll see that they satisfies the axioms of a Hopf algebra, plus the condition that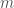 and
and 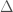 are commutative. Moreover, these axioms are self dual, in that, if you take the transpose of al these maps, you’ll get another such class of maps, with
are commutative. Moreover, these axioms are self dual, in that, if you take the transpose of al these maps, you’ll get another such class of maps, with  and
and  changing places, as do
changing places, as do 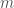 and
and 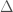 . Canonically, this operation replaces
. Canonically, this operation replaces 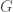 by its character group.
by its character group.
If we no longer insist that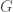 is commutative, then everything stays the same, except that
is commutative, then everything stays the same, except that 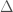 is no longer commutative. Symmetry suggests that we should also drop the assumption that
is no longer commutative. Symmetry suggests that we should also drop the assumption that 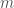 is commutative; this gives us the axioms of a Hopf algebra!
is commutative; this gives us the axioms of a Hopf algebra!
Of course, maybe you already know all of this. (Your question wasn’t very specific.) I don’t know where you should go to read about the structure theory of Hopf algebras, but Noah, Scott M. and our frequent commentor Greg Kuperberg probably all have ideas.
Just wanted to make sure that John Mangual, and everyone else who was interested, saw Charles’ great series of blog posts on Gromov-Witten theory. He provides a lot of the technical algebraic details to go with AJ’s great big picture answer.
This is great. Now I can say it in one sentence. A Gromov-Witten invariant is an integral of a product of cohomology classes over the Moduli space of marked curves.
Actually I have no idea what I just said.
Some implications: cohomology classes can be multiplied, cohomology classes can be integrated, the moduli space of [stable] curves can be parameterized.
The moduli space is a space of curves up to isomorphism as compact Riemann surfaces. So M(g,n) is some 3g – 3 + n manifold. We can also talk about the moduli space of stable maps from one of these curves to a ‘nice’ (I have no idea what ‘nice’ means here) complex variety.
Thus, a cohomology class is just a function which takes a stable curve to an integer. I’m not sure how this is integrated.
John, need to go ONE step further…it’s an integral over the moduli space of stable maps from marked curves. Also,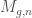 is only an orbifold: it can have singular points, but they aren’t THAT bad.
is only an orbifold: it can have singular points, but they aren’t THAT bad.
As for the niceness conditions…we’re loosening them rapidly. The cohomology classes form a sort of ring (not quite commutative, but we only care about the commutative part, in general) and then integration is a function that takes classes to numbers (strictly, rational numbers, but they’re integers in the cases I talked about). What the cohomology classes are, really, are things representing collections of curves, and integration takes these collections to integers, and generally it’s just counting isolated points (it kills any positive dimensional families of curves). Let me know if there’s more I can do to clear it up.
As for some of your other questions, here’s the answers that I can give:
Sheaves are wonderful bookkeeping devices. The first use that most people see for them is keeping track of locally defined functions and when they can be patched together to functions on larger sets. Then they turn out to have all sorts of amazing properties. Basically any time you have local information and want to know about when you can patch together, sheaves pop up, including vector bundles and, more generally,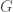 -bundles. The formalism is very flexible and can represent a lot of different things, which is why people care. As for perverse sheaves, I know nothing.
-bundles. The formalism is very flexible and can represent a lot of different things, which is why people care. As for perverse sheaves, I know nothing.
For what a scheme is, I wrote about that here, hopefully you’ll find that helpful.
Now, earlier, you asked about Schubert Calculus…I’m planning some posts on that, once I dig my way out of the pile of Gromov-Witten, Donaldson-Thoman, and Stable Pair papers I’m piled under, but though it’s very ad hoc and doesn’t give much general detail, I did write this which involves some Schubert Calculus.
Yeah I guess I’ve asked a lot of questions over time. For now I guess I don’t really have anything to ask about sheaves so I’ll focus on the enumerative stuff.
It seems like Schubert Calculus and Gromov-Witten theory both count things.
Schubert Calculus seems to revolve around the cohomology of the Grassmanian G(n,k) – and somehow Chern classes come into play here too. So there seems to be two problems: understanding the cohomology ring itself (which *must* be well understood by now) and then learning how to translate enumerative problems into this language.
1) What is the structure of the cohomology ring of the grassmanian G(n,k)?
2) How can I use this structure to answer enumerative problems about hyperplanes? (In other words, how to encode real geometric problems in terms of cohomology ring?)
I ask these questions because these are probably well understood objects where good explanations may appear in a book somewhere. On the other hand, I like talking to real live people too. You can’t ask a book to clarify.
For Gromov-Witten theory I have basically the same questions. I imagine that it’s probably a feat if you use this theory successfully to compute things. At the end of all this investigation, I just want to have a few realistic exaamples of gromov-witten calculations that I can hang up in the living room next to the television or by the window. Or maybe it’s more like a household appliance…
The Schubert Calc stuff will be covered in a post on my blog in the near future. As for GW theory, well, it counts curves, though it’s current and being studied rapidly right now, so we’re still pushing what exactly it can count and under what circumstances. As for actually counting things with it, it’s not as bad as it sounds. For instance, there was my post on Kontsevich’s Formula, but also there’s a relation to my most recent post, the one on the Clemens Conjecture. The number of rational curves in a quintic threefold is predicted by the GW invariants, which can be calculated via the conjectured Mirror Symmetry. Sadly, we’ve not yet proved that these are the right numbers, but my understanding is that they are for as far as we’ve managed to show that the number is finite.
I’d like a new El Naschie post summarizing latest developments.
Anyone know of any expository sources on Deligne’s paper “La Categorie des Representations du Groupe Symetrique S_t, lorsque t n’est pas un Entier Naturel.” The combination of lack of pictures, lots of technical category theory, and being in French is a bit intimidating (any two of those three I’d be fine with).
The toric variety post (request 48) is now up.
There is now a sequel to the toric variety post. At the moment, no further posts on this subject are planned.
Hi,
Here are two papers by F. Knop extending Deligne’s construction (I saw him give a talk about this in Göttingen):
http://arxiv.org/abs/math.CT/0605126
http://arxiv.org/abs/math.CT/0610552
They match your criterion by being in English (I would have preferred the pictures…), the first one is purely technical but only 6 pages short, the second is more reader-friendly.
John, if you’re still interested, I’ve just made the first post in my series going to schubert calculus. It’s here.
Noah — I’ve been reading the Knop references Peter Arndt cites in 78, and I have a pretty good idea what Deligne is doing. I’m working on a post now.
Excellent! I looked at those papers for a little while too, and they look very interesting. In particular when I’d looked at Deligne’s paper I had no idea that his construction had a chance of applying to more than just the case of S_n. David, I look forward to reading what you have to say about them. Thanks Peter for the links! I may later on have a follow-up about another way to think about S_t with more pictures.
A request for a posting about math jobs:
It bugs me that so many of the positions on the math jobs wiki are simply listed as “filled”. Of course it’s a touchy subject if someone is interviewing or has gotten an offer or whatever. But once someone has accepted a position, I don’t see that there is any controversy left at all, either for the candidate or for the department. Could people post their own names when they get a job; or could departments post who they have hired?
One of my main motivations in setting up the math jobs wiki is to let people know, if they didn’t get hired somewhere, who got hired instead. I think that people have the right to know why they didn’t succeed.
So I have a torus and some metric on it. By uniformization it is conformally equivalent to a flat torus with a certain aspect ratio. Is there any way to compute it from the metric alone?
More generally, given a surface and a complex structure on it, how can I compute the moduli space representative (or even just the Teichmuller coordinates) from the metric?
This blog already contains quite a bit of useful career advice, and it would be great to see here a discussion of how one gets to give *invited* lectures at the conferences. I hope one could learn more than the standard replies like “do the outstanding work, and the invitations will follow…” Many thanks in advance!
This is a request to Ben Webster on the L’Affaire El Naschie post. You are not keeping it up-to-date; one of the first links is broken, because Baez has pulled his stuff down. Would you please send people over to El Naschie Watch with a note PREPENDED to the article rather than just the existing note at the end of the comments? This will save everyone a lot of frustration since you are not keeping broken links up to date, and don’t want to be the go-to guys for El Naschie information anyway. I keep an archive of the stuff Baez pulled down, and much much more. Thanks! –Jason
Just a quick head’s up that the Tricki (http://www.tricki.org/) is now online.
A typographical question: what is the name of the font used in old IHES publications, e.g., EGA? Is there a tex style file that reproduces that style?
DD: it’s been a while since I’ve looked at EGA, but if I remember correctly the font it used was called a typewriter.
More seriously, if I’m correct I think the most common typewriter typeface was very closely related to Courier, if it wasn’t Courier itself.
As regards Post #91:
Dear John,
I think you’re confusing EGA with SGA; here is an example of the typesetting in question http://archive.numdam.org/article/PMIHES_1960__4__5_0.djvu
Sorry, I don’t have a viewer capable of opening DjVu files. Maybe you have a nice, normal PDF around?
Here’s the PDF version of EGA I (29 mb file!)
Click to access PMIHES_1960__4__5_0.pdf
No time like the present to make a change (here). Scans of books tend to gigundous in PDF format. DJVu’s are much smaller.
DD – I reposted your question at a blog where a lot of typesetters hang out. The best guess is Baskerville.
DD and David — It is indeed a version of Baskerville. New IHES publications are typeset with TeX using SMF Baskerville, which was developed to copy the typesetting of classic IHES publications. See http://omega.enstb.org/yannis/pdf/article-gut99.pdf for a description of it (in French).
I have always thought that the IHES typesetting is by far the most elegant of any mathematical publisher. One can buy the font they use (for a LOT of money!), and several times I’ve considered buying it for my own use…
I’m not a geometer but have an interest in art. I have long wondered how I can make animated art pieces (computer, viewable) using flashing lines of dots like the old Bijou movie marquees with bulbs going off and on to create moving dots and lines. One idea would be to start with a picture (e.g Mona Lisa) and have it break up into lines and rotating lines of dots and get random for a while, then reassembling every now and then to reform the picture. Does anyone have any hints as how to do this (and many other constructions)? Thanks
This is test, ignore.
Hey Nathan,
Your recent comments got stuck in our spam box. I unlocked this one, and one of your three comments on Ben’s post.
Sorry!
I’d like to know what ind varieties (schemes) are and how one thinks about them / works with them, and also why they are necessary, i.e., why are things like affine Grassmannians not varieties / schemes?
In any category, an ind-object is simply a diagram of objects. You would like to take a limit of this diagram but such a limit may not exist in the category. So morally speaking, just pretend the limit exists and call it an ind-object.
The simplest example of an ind-variety that I know is the limit of the sequence of inclusions . The affine Grassmannian is of approximately the same form, as a sequence of inclusions of subvarieties of increasing dimension.
. The affine Grassmannian is of approximately the same form, as a sequence of inclusions of subvarieties of increasing dimension.
It is easy to see that the resulting object is too big to be noetherian, to see it can’t be any scheme, as always one reduces it to a corresponding question in ring theory. I believe for such an ind-variety to be a scheme, one would have to have a ring together with a saturated chain of prime ideals
together with a saturated chain of prime ideals 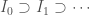 with the property that all maximal ideals
with the property that all maximal ideals  contain some
contain some 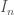 . I claim that such a setup is impossible (Exercise).
. I claim that such a setup is impossible (Exercise).
If one wants to think about ind-varieties, note that you can always make sence of the functor of points of an ind-variety, so you can think about it in this way as an algebraic space (and of course by Yoneda’s lemma, the functor of points tells us everything). In practice, every time I’ve been working with the affine Grassmannian, all the action has been taking place on a bona-fide finite dimensional subvariety, so I’ve been able to get away with only knowing some theory of algebraic varieties. I’m not sure how typical this experience is.
Peter: with the affine space example, wouldn’t the limit be Spec of a polynomial ring in countably many variables?
Spec of a polynomial ring in countably many variables can be identified with infinite tuples of elements of our field. The example I am trying to mention is a vector space of countably infinite dimension, which is strictly smaller (ie only finitely many coordinates nonzero). Every point in this space is required to lie in some
is required to lie in some 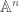 .
.
Is it too soon to make some predicitons about the 2010 Fields medals? Or at least talk about who you think deserves one? Last time around I feel like everyone was expecting Tao and Perelman to get medals, whereas this time maybe there aren’t analogous clear frontrunners. Maybe I’m wrong?
I’d be very interested in learning the proof of the Mitchell embedding theorem, and more about category theory in general (especially tensor categories)?
Younger mathematicians than I sometimes lament the
solving of so many big problems in the 20th century, but
there are significant problems left over at the interface of
representation theory and algebraic geometry. One which
has been somewhat neglected since the important work of
Henning Haahr Andersen (following up his 1977 MIT thesis) concerns higher cohomology of line bundles on flag
varieties in prime characteristic. Though Kempf’s vanishing theorem (which plays a major role
in Jantzen’s book “Representations of Algebraic
Groups”) shows that the lowest and highest cohomology
behave as in the characteristic 0 Borel-Weil Theorem,
the intermediate degrees require more subtlety. I’m still
convinced, as in my 1986 paper in Adv. in Math. and my
more detailed follow-up in a 1987 JPAA paper,
that the answer requires Kazhdan-Lusztig theory for the
affine Weyl group (of dual Langlands type). I worked out a
lot of unpublished details for the rank 2 case of G2, but in
general one needs higher-powered methods.
This problem is not superficially close to the study of
simple modules (Lusztig conjectures for groups and Lie
algebras), but seems intrinsically challenging and in
need of fresh thinking. In a modified form the parallel
problem remains unsolved for quantum groups at a
root of unity.
Full flags are indeed probably intrinsically challenging. But does someone know at least the cohomology of obvious vector bundles on Grassmannians? Here, obvious means bundles built from tautological bundles using multilinear operations. For projective space, you can work out the cohomology of such bundles in arbitrary characteristic
by just starting with the Euler sequence and then playing with long exact sequences. I’m curious whether anyone has had the patience to do the same for Grassmannians or whether it is known that the answer is not characteristic free.
Chris: I haven’t checked this carefully, but I think all the “obvious” vector bundles you mention are pushforwards from line bundles on the flag variety (or direct sums of such pushforwards)? So cohomology of these vector bundles is related to cohomology of the line bundles Jim Humphreys asks about by a spectral sequence.
My guess (without much justification) is that working with full flags is easier than working with Grassmannians here, and that transforming between the two problems is easier than solving either one.
I think that Griffith’s example of characteristic dependence http://www.ams.org/mathscinet-getitem?mr=573481 pushes down to an example on . Specifically, Griffith’s first example (his Theorem 3.1) is that the line bundle
. Specifically, Griffith’s first example (his Theorem 3.1) is that the line bundle  has nonvanishing
has nonvanishing  on
on  in characteristic
in characteristic  , but not in characteristic zero.
, but not in characteristic zero.
I didn’t dot every i and cross every t, but I thought a bit about what happens when you push down to
down to  . It looks to me like you get
. It looks to me like you get  , where
, where  is the tautological
is the tautological 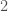 -bundle. It looks to me like Griffith’s resolution (1) pushes down to a resolution of this vector bundle; and the long exact sequence in cohomology behaves exactly the same was as in Griffith’s paper.
-bundle. It looks to me like Griffith’s resolution (1) pushes down to a resolution of this vector bundle; and the long exact sequence in cohomology behaves exactly the same was as in Griffith’s paper.
Sadly, Griffith’s paper doesn’t seem to be online and I’m too lazy to retype it. But you can take this as a challenge: compute the pushdown of to
to  , and its cohomology.
, and its cohomology.
UPDATE (December 9) I just looked back at this answer and found it had a very confusing typo in it. In the first paragraph, last sentence,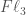 used to read
used to read  . Sorry about any confusion that caused!
. Sorry about any confusion that caused!
David and Chris,
I don’t want to overload the detail, since the history is
 such as Grassmannians play an essential
such as Grassmannians play an essential
complicated. But Larry Griffith (now teaching computer
science at nearby Westfield State College) did a Harvard
thesis with Mumford which got other people thinking,
including Seshadri and then Andersen, etc. Griffith’s
results/methods were awkward in retrospect, while
Andersen made more creative use of Demazure’s
“simple” proof of Bott’s Theorem. Here the varieties
role in the exact sequences, but in characteristic p all
gets complicated after the projective line case. The
vanishing behavior of cohomology is still not settled
beyond the misleadingly nice top and bottom degrees
treated by Kempf. And the representations are usually
far from irreducible, which drew me to the subject.
The papers by H.H. Andersen are the best resource,
and many are available online. (I have copies or reprints
of everything including Griffith’s thesis.) Footnote:
Henning’s family name is Haahr Andersen, under H in
the Aarhus phonebook, but the pronunciation in the U.S.
is awkward. His wife’s family name was Andersen, which
caused difficulty with the driver’s license people in NJ
when they spent a year at IAS.
but the pronunciation in the U.S.
is awkward.
Any of you curious about what this means can email me for explanation. This is a family blog, so I won’t say anything more.
I had known that in characteristic zero, tensor products of Schur functors of tautological sub and quotient bundles on Grassmannians were pushed-forward from line bundles on full flags, but did not realize that this was the case in all characteristics since I had not known Kempf vanishing (higher cohomology vanishes for line bundles labeled by a dominant weight).
However, these particular line bundles on full flags are of a very special sort, where the weight splits into two parts, one of length k, dominant for GL_k, and the other of length n-k, dominant for GL_n-k. Is it the case that cohomology is not known for such line bundles?
Chris,
I’m not sure offhand about what is known in this special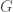 -module structure in the case of line bundles remain
-module structure in the case of line bundles remain , Lusztig’s old conjecture on
, Lusztig’s old conjecture on
case, but I should have emphasized that there is a certain
amount of information about low ranks and some other
cases in the literature (just very little general theory).
Probably the best-informed people are Andersen and
Jantzen at Aarhus, Donkin at York. Also, I should emphasize that standard computational methods require
some study of cohomology of more general vector
bundles. But the vanishing behavior and
largely unknown, apart from the antidominant/dominant
line bundles where Kempf plus Serre duality give the
classical vanishing behavior and where the modules are
Weyl/dual Weyl modules still being actively studied
(for large enough
composition factor multiplicities via KL polynomials for
the dual affine Weyl group). In other situations the
early work of Andersen shows mainly in low
ranks how nonvanishing can occur in more than one
degree and how the module can even be decomposable.
Generically the modules are twisted versions of Weyl
modules, but otherwise get messy to study. Probably
the vanishing can’t be well understood apart from module
structure and KL theory, except in isolated cases.
Hey,
I was wondering if somebody would be interested in writing up a discussion of the transition from “classical” algebraic geometry to “modern/Grothendieck” algebraic geometry. To be a bit more specific, I’m spending the summer working through the fundamentals of scheme theory, but to be quite honest, I’m having a lot of trouble understanding the revolutionary nature of the French school of algebraic geometry. I guess I’d be interested in seeing a post that gave a concrete example of a particular problem, as interpreted in the classical sense, and how it was reinterpreted in the modern sense.
Best,
Anon
A late followup to Jim’s post on cohomology of line bundles on flag varieties in positive characteristic:
A closely related problem is that of finding minimal free resolutions (or just Betti numbers) for determinantal ideals in positive characteristic. Jerzy Weyman explains the connection, originally used by Lascoux in the 70s to find the resolutions in characteristic 0 (modulo a minor gap long since filled), in his book. As far as I know, the most recent reference on positive characteristic resolutions is still the semi-posthumous paper of Buchsbaum and Rota about all their various not particularly successful attempts.
This version of (part of – if i remember correctly not all the line bundles are needed) the problem is a little easier to approach in that one can quite easily get an answer from your favourite commutative algebra package in small but nontrivial examples, though it seems highly unlikely that one can find much of an answer by staring at the computable examples.
In reply to Anon (comment 113),
Until such time as the post you requested appears, you might like to look at Mumford’s wonderful book “Lectures on curves on an algebraic surface”, or at least the introduction. You could also read Mumford’s article “Picard groups of moduli problems”, or again, at least the introduction.
Without leaving the confines of Hartshorne’s book, you could read his discussion of the Hilbert scheme, or of the Jacobian of a curve, or his proof (which is Grothendieck’s proof) of the theorem on formal functions, and its corollaries: Stein factorization and the Zariski connectedness theorem. The first two illustrate Grothendieck’s conceptualization of moduli problems in functorial terms, and the latter illustrates the way Grothendieck uses the interplay of schemes, formal schemes, and cohomology to make arguments. Although the latter predates Grothendieck, the theorem on formal functions and the connectedness theorem were among the most difficult results of pre-Grothendieckian algebraic geometry, and the way they get reformulated and reproved from his point of view is worth understanding.
One more suggestion: in Mumford’s red book, he discusses Zariski’s main theorem, and how Grothendieck ultimately reinterpreted and generalized it; this is another good illustration of the power and focus of Grothendieck’s view-point.
Not strictly a request, but some of you may take an
interest (morbid or otherwise) in the ICM 2010 speakers
lists just published. The selection process emphasizes
“international”, since it would be easy to fill up the
program with people currently based in the US, but in
any case it’s an interesting cross-section of people who
do know something about their subject. (In case you are
planning to attend the congress in Hyderabad, take the
visa requirements seriously. I was once supposed to
go to a conference there and followed all the rules, but
my passport with visa came back too late. Armand
Borel once tried to expedite a visa by taking his passport
to the NYC consulate, where it was tossed onto a huge
pile of other passports awaiting action.) Caveat emptor.
http://www.icm2010.org.in/speakers.php
I just arrived to begin a postdoc at Princeton, and have been discussing with some of the other junior faculty the possibility of an informal trans-disciplinary seminar among postdocs in the department. I’d love to see what the writers and readers of this blog think are the important ingredients of such a seminar. Since the goal would be to have it be fairly broad, it couldn’t be a research seminar as such. I want to have it mainly as a way to build community and to foster new mathematical and personal interactions. But I also see other benefits, such as more practice in talking about your work to a general audience before having to do it on the job market.
I’ve been told that a few years ago MIT had something called the “undistinguished seminar” which the postdocs there at the time found to be a lot of fun. What made it work? Are there other models out there? We have already decided that a bar outing after the seminar meets is an important ingredient. Other ideas?
Thanks!
Sue
This is not exactly a request… I just learned that Israel Gelfand is no longer with us. R.I.P.
In trying to construct a set of all the real numbers from 0 to 1, I was able to create an infinite set which appears as having recursive, fractal-like nature, so that between any two specified numbers in the set, I could specify an infinite set of further numbers that are, recursively, mirror images of the previously specified ones.
So far, this is all expected, yes yes of course. This set is what Cantor’s diagonal predicts, I understand.
A problem I wonder about is that this infinite set ought to be arranged into a sequence, and from that sequence a Cantor’s diagonal ought to be constructed, and from that diagonal, we ought to find a number that is not already specified.
But I already showed that between any two numbers, there is a specified infinite set of all the smaller numbers that lodge between those. That is, the diagonal so constructed is already acknowledged or recognized, theoretically.
The way out of this difficulty would be to say that the exhaustive list could not be arranged in order of the counting numbers (as Cantor assumed?); or that a diagonal could not be constructed (as assumed?); or both.
I have written my speculations in more rigorous form than what I just told you. Would anyone like to help me sort this out? Either I’m making a simple error or I’m overthrown the nondenumeracy principle, which seems unlikely.
Bruce, your error seems to be in assuming that the ordering of numbers in the list has anything to do with the ordering the set inherits from the real numbers. Cantor’s diagonal argument doesn’t say anything about a new number being between a fixed pair of numbers. It simply says that if you have ANY countable set of real numbers, there will be a real number that is not in the set.
By ‘countable’, do you mean finite? The key to my formulation was that for any finite power expansion of my basic set, (details available), that there would be an easily discoverable real number not in it; but that if the set were raised to one higher power, that new set would include the exception. This would be true for all finite power expansions of the basic set.
So I ask, what if we make the power “infinity” rather than any finite n? The counter-proof number would have to be included because infinity plus one is still just infinity.
But it looks like what you are saying is that when the power is infinity, that the set is not countable. Which is what I was speculating–that is, this is what I meant when I said that an exhaustive lives could not be arranged in a countable fashion.
So what I came up with is a procedure for generating a non-countable set of real numbers. In which case Cantor is not making a claim against it.
The basic set is (.0 .1, .2, …, .9), with ten members to one decimal place. If we ask if there are any more real numbers that exist, we merely go to the hundredths place. So the set is expanded by a power of n+1. If n=1, there are ten members, if n-2, there are 100 members, and so forth. The exception would be at a power of one greater than the existing finite power n.
So I supposed that if n were infinity, that there would not be an exception number, that all the nonrepeating and repeating numbers would be in this set, that the set would be fully dense, and no diagonal counterproof could be constructed. I was supposing however that the members of this set could be arranged to correspond to the counting numbers, and a diagonal constructed. It seems then that the set I specified (where n-power is infinity) is not, in fact countable. I’m a little hazy on what makes an infinite set countable or not countable.
Did I understand you right?
Bruce, I am having difficulty understanding what you are saying, in part because your use of terminology does not conform to the conventions of modern mathematics. As far as I can tell, you are constructing the set of all decimal representations of all real numbers between 0 and 1, and Cantor proved that this set is uncountable. If you are having trouble with the concept of countable set, I think it would be best if you were to read about it, and familiarize yourself with standard techniques for proving countability and uncountability, before attempting to find contradictions in set theory.
My thought is that his problem lies in the same place as something that went on the arxiv awhile back. The number of paths through the tree he’s constructing isn’t countable, the finite paths are, and those only give the reals with finite decimal expansion. There is a bijection of the reals with the paths through a tree branching ten times at each node, but that tree isn’t countable.
Thanks to Scott and Charles. If after this message, I still am missing something about countable sets (or other points), I promise to go read up on it. But Charles seems to have gotten my point. The tree is infinite in extension, and the finite paths are all and only those paths that terminate at zero, i.e., out of every branching, only one of ten would give rise to a repeating zero (or any other numeral) in every subsequent branching. In this way the infinite tree allows for every terminating, nonterminating, repeating and nonrepeating decimal. I understand *this set*, represented by the entire infinite tree, to be the entire real number set from 0 to 1 (inclusive, since 0.000… and 0.999… are two included branches).
From what I can gather, the numbers of this tree are a set of higher cardinality than the counting numbers. In which case, no contradiction of set theory is indicated.
Here’s the big but:
As Charles touched on, my interest was in representing the non-finite decimal expansions that would lie between any arbitrarily chosen finite decimal expansions. It is this group of non-terminating and nonrepeating numbers that exist in the infinite branching tree, and that I want to be part of the construction of a Cantor’s Number.
A sequence of decimals could be constructed by this tree, upon which a non-appearing diagonal ought to be constructed. The first ten items are 0.000…, .1000, .2000, … .9000, followed by the next branching of 0.000…, 0.01, 0.02, thru 0.99, followed by the next branching at the thousandths place. Those paths that terminate with an ever-repeating zero could be entered merely once, which is one out of every ten at every iteration. There seems no theoretical hindrance to stacking the ever greater-sized sets into a list, upon which a diagonal could be constructed. At any arbitrary point, the stacked branches are countable, *but the entire infinite expansion of branches ought to be countable* as well. The number of branches to be sequenced is itself infinitely large, and a larger infinity than the counting numbers (as far as I can tell). But even so, it seems to me that this entire sequence of stacked branches could be set into a one-to-one correspondence with the counting numbers. From which a Cantor’s Diagonal could be constructed, which would not appear in the stack itself. Even though the stack itself is exhaustive of all decimals from 0.0 to 0.9….
You’re missing the point. It’s not exhaustive. The nodes of the tree are in one-to-one correspondence with finite expansions. The things that are in correspondence with the reals are the PATHS from the root all the way up the tree.
Do you mean that the set of all paths, an infinite set, is not exhaustive?
You said, Each path represents a decimal expansion, which include all the reals. I’m pointing out that there are infinitely many paths, the number of paths being a function of the number of nodes, which are themselves a function of the decimal place out to the right, which themselves correspond to the infinitely many counting numbers. These paths include all the nonterminating and nonrepeating decimals, plus the repeating, plus the finite (terminating) decimals. These paths may be stacked. From this stack, a Cantor Diagonal be drawn.
Isn’t that what I said?
Each node denotes nine new decimal additions to the previous number and one additional zero, which indicates the termination of the previous decimal expansion. There are infinitely many nodes going out (covering the nonterminating numbers), and the nodes are themselves items of a set corresponding to the next-decimal-place out. There are infinitely many sets of nodes going out, corresponding to the decimal places 1, 2, 3…, each node having ten members (one member of the terminal branch and nine unique decimal additions to the previous decimal on the left side of the node), and the number of nodes going down (10 at the first, 100 at the second) is a function (ten to one) of the n-number of the nodes on the right.
Between any two adjacent branches, at any given node, there are no decimal numbers that can fit between them, except for the decimal expansions to come, on the right; of which there are infinitely many decimal expansions which exist on the right.
The problem (for me) now becomes, can the infinitely many paths (=branches) be stacked? and if stacked, can a Cantor’s Diagonal be constructed?
No, the infinity of paths cannot be put into bijection with the naturals. That’s the point. The number of paths of length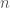 is
is  . So the paths with length
. So the paths with length 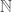 are of cardinality
are of cardinality  , the cardinality of the power set of the naturals, which, by diagonalization, cannot be counted.
, the cardinality of the power set of the naturals, which, by diagonalization, cannot be counted.
Thank you. Your explanation is coherent, and you’ve pointed me in the right direction, i.e., to study these concepts more properly.
I did understand that the power set of the natural numbers is of a higher cardinality than the naturals themselves–but I didn’t think that the constructed number tree was it. My version of the Power Set wore glasses and came from Smallville–it didn’t look like the tidy construction I ran across in the textbooks.
I stopped by a mathematician at my local college (MIT, lol) (I’m outside of Cambridge, MA), to run an earlier version of the theory by him, and he said that I proved, by a back door, what Cantor did directly. I didn’t understand the details but felt too embarrassed to take up more of the good professor’s time.
Well, it’s not quite the power set that’s showing up, just something with the same cardinality (ok, so I guess we can’t legitimately tell them apart in some senses, but anyway…). To read further, any book on set theory will have a section on cardinality, and I know that a few are available online, or from Dover (and thus, are cheap).
One more smallish concern/question/problem. Cantor constructed a decimal list based on a grid of all the numerators of the natural numbers moving to the right, and the denominators likewise taken from each of the natural numbers moving down. This gives us every possible ratio, 1/1, 1/2, 1/3…2/1, 2/2, 2/3, … From this grid, we eliminate all the duplications, and move diagonally, we can construct an exhaustive list of decimal numbers. From this exhaustive list, we then construct the Cantor’s Diagonal, which proves that there are more numbers even yet.
Why isn’t Cantor’s grid the same cardinality as my tree, and as such, the same cardinality of the Power Set? I surmise that if we restrict the construction of the Cantor grid to ratios that have a numerator less than or equal to the denominator, we should have a result identical to my tree, except written in a different order.
There is no Cantor diagonal available for my tree, being of greater cardinality than the natural numbers. Yet the tree should exactly duplicate the content of the grid I describe here with the restriction on numerators (preceding paragraph), which DOES provide a Cantor diagonal.
Any ideas?
No, that list gives precisely the rational numbers. It doesn’t include . And it is the same cardinality as your TREE. You’re ignoring the fact that the real numers aren’t points in your tree, but PATHS IN YOUR TREE.
. And it is the same cardinality as your TREE. You’re ignoring the fact that the real numers aren’t points in your tree, but PATHS IN YOUR TREE.
No, I’m insisting that the paths are the numbers. The points in the tree would be place markers for finitely terminated numbers.
The understanding that I’m working with–hidden assumption?–is that a decimal is a fancy way of writing numbers, be they rational or irrational. An irrational such as pi is 3+(1/10) + (4/100) +… and though it may not be terminal, it is the relationship of so many digits in the numerator and so many places in the denominator. That is all that it means to WRITE something as a decimal expansion. And we write irrational numbers as decimal expansions with exactly that understanding.
The paths in the tree include all the irrationals. The path of pi would exist. But I do see that the tree has this advantage over the Cantor Grid: irrationals appear in the tree via their paths, but in order for an irrational to appear in the grid, there would have to be a determinate (i.e., terminated) numerator over (for a decimal) 10 to power (appropriate to the numerator). Pi has no final digit, hence cannot be represented in Cantor’s Grid, but it does have a determinate path in my tree, so it IS represented therein.
I’ve been insisting, up to this point, that a decimal expansion of any kind would be of the form (numerator to n places)/(denominator to n+1 places), which would make it a ratio. I thought–and still don’t see why not–that for an irrational number to exist as itself, it has to have a determinate content, SOME specific numerator over SOME specific denominator; and could NOT be “roughly” something. If it IS something, it is NOT anything else.
I thought that “something” has to be a ratio of numerator to denominator, determinate yet undiscoverable. Determinate meaning “it is what it is, and not something else.”
Here is my request.
I would like to be able to search the arxiv for all papers citing any given paper.
@Peter: citebase.org does a reasonable job of this, at least within the arxiv world.
@Peter Also, MathSciNet offers this option. Of course, the citing and cited paper must be in MathSciNet and the citing paper must be fairly recent. But, within those parameters, I find this extremely useful. See the “from references” link in the upper right.
@Peter: Google Scholar is my go-to application for this. It’s not perfect, but it’s the most complete I know.
Bruce: there are more paths than nodes in your tree. That’s the point. But at this point you’re repeating yourself, and so am I, because you’re not listening to me. is NOT a node in your tree, but it IS a path in your tree. The PATHS of your tree are in correspondence with real numbers, yes. But the NODES, which are the things that are in correspondence with the naturals, are not. Thus, you do not have a counterexample to Cantor. Period. Full stop. There was a crackpot paper on the arxiv a bit over a year ago, it’s here. You’re making EXACTLY the same argument, and EXACTLY the same mistake. Just to make sure you don’t leave convinced that it’s right, here’s some info:
is NOT a node in your tree, but it IS a path in your tree. The PATHS of your tree are in correspondence with real numbers, yes. But the NODES, which are the things that are in correspondence with the naturals, are not. Thus, you do not have a counterexample to Cantor. Period. Full stop. There was a crackpot paper on the arxiv a bit over a year ago, it’s here. You’re making EXACTLY the same argument, and EXACTLY the same mistake. Just to make sure you don’t leave convinced that it’s right, here’s some info:
It’s been posted for a year, and has zero citations.
Also, it received some blog attention to be defeated thoroughly, I’m just going to link to Good Math, Bad Math, where Mark did a MUCH more thorough job explaining why this approach fails than I can in comments.
You’re misreading me or I’m mistating myself, because *you persuaded me*. Hard to believe in academics, but you won me over by the clarity of your argument. I actually stated in my own words why you are right, in the second half of my statement.
Perhaps confusing was my description of an irrational as the sum of ratios, i.e., an irrational can be read from left to right as one ratio added to another ad infinitum, but cannot be stated as a single ratio, because there is no termination. I stated my misconception first (in medieval disputation format) and proceeded to show its inadequacy, by restating *your* clear argument.
Perhaps interesting to those with a bent to metaphysics, this discussion is similar to one about the possibility of a physical infinity of material space and objects in space.
Why was I confused? It is because when I first learned these concepts, they were presented as “received truths” to be heard and learned and integrated into the rest of knowledge. That’s fine, but many more connections need to be made, e.g., these here, before the knowledge gained becomes usable. Howard Gardner reports testing a set of newly-graduated Physics majors and asking them physics questions framed in ordinary, non-technical language. They would inevitably give the common sense, Aristotelian-physics answer rather than what they learned in theoretical physics.
(A typical question is, if you drop a rock out of a moving car or airplane, where will it land?)
Thank you, my friends.
@Peter: You can. For example, your paper 0211126 has been cited in 0502278. This probably won’t surprise you, but hopefully it will surprise you that I was able to find this out in about 20 seconds.
@135,136,137
Thank you for your useful suggestions. I have used mathscinet in the past for this, and the question was prompted by a desire to find more recent preprints than appear in mathscinet. I’ve had a quick look at citebase and google scholar and can’t yet see one service as being obviously better. I wonder how these services are produced, because they have data from arxiv preprints, but the “search for all papers citing this one” does not get to the most recent arxiv postings.
@Andrew: I didn’t actually write 0211126. I’d like to apologise to Peter R W McNamara and anyone confused about my identity for failing to put any distinguishing middle initial in my comments on this or other blogs to date.
I know of particular cases where citebase has missed references to my papers. Google Scholar I haven’t had that problem with, though it does pick up some things which are not papers.
@Peter: Okay, so I didn’t do a thorough check on names! However, I hope you got my point that it is possible to take someone’s name, look up their papers on the arxiv, and then look up which papers on the arxiv cite those. The fact that I could do that in about 20s and clearly know absolutely nothing about the people or papers concerned should at least tell you something!
However, clearly trying to give a “teaser” didn’t work. The arXiv now has a “full text” search facility. It’s labelled as “experimental” but as it goes from the direct data, it’ll be more up-to-date than citebase, and as it is just the arxiv data, it’ll be more reliable than google scholar.
To use it, click “Advanced Search” from an arXiv page.
Could you post a link to this my blog post with an interesting lattice theoretic conjecture to raise the attention of mathematicians to that problem:
http ://portonmath.wordpress.com/2009/11/02/exposition-complementive-filters/
@porton 145: No, sorry.
In particular, we don’t link to crackpots, and you’ve already scored 20 points. Rule 21, in particular.
Hello, do you guys feel like providing some stats on MathOverflow? It’s been running for roughly two months now, so there are probably interesting trends emerging. For instance I’ve counted just now 525 “active” users (i.e. those strictly above the basic 11 points, so with at least one upvote). Questions one might ask: is that number what you expected, how did that evolve, how many more will join at this rate, what’s the ratio between senior folks and grad students, is it easy enough to locate previously answered questions, and so on…? That would be a great discussion to have IMHO, cheers.
Does anyone have an opinion on Alain Badiou’s use of set theory? Is there anything interesting mathematically there? Also could anyone shed any light on the comment in the Wikipedia article link text that says:
“This effort leads him, in Being and Event, to combine rigorous mathematical formulae with his readings of poets such as Mallarmé and Hölderlin and religious thinkers such as Pascal.”
I’m not impressed by what a little Googling shows, but I tend to be unimpressed by philosophers.
Perhaps someone would like to talk about the Etale topology and why it’s so much cooler than the Zariski topology?
Please indulge me with this. What would the reciprocal of an irrational number be? That is, if we can’t specify pi minus 3 to a finite conclusion, say; and all real numbers must have reciprocals; how can we specify its reciprocal?
Thanks.
Let’s say your real number is specified as the limit of a sequence of rational numbers. For example, is the limit of
is the limit of  ,
,  ,
,  etcetera. Then
etcetera. Then 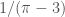 is the limit of the sequence of reciprocals:
is the limit of the sequence of reciprocals:  ,
,  ,
, 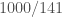 , etcetera.
, etcetera.
Hi, i just started graduate study after a year of work, may i ask this simple question.
Why is it that if the continuous functions fn, defined as fn(x) = n if 0<x<1/n and fn(x) = 0 elsewhere (so fn(x) approaches o for every x). Why is it that the integral S of fn(x) from 0 to 1is equal to 1. I'm quite confused of the exact reason, since fn(0) =1 and fn(1)=0 too. Although the lim fn(x) before between zero to before 1, seems to be 1.
Sorry, just missing some of my calculus lessons perhaps. Many Thanks in advance for your response & advice. :-D
because :)
:)![[0,1]](https://s0.wp.com/latex.php?latex=%5B0%2C1%5D&bg=ffffff&fg=444444&s=0&c=20201002) where it is nonzero, i.e. the integral between 0 and 1
where it is nonzero, i.e. the integral between 0 and 1
and that’s the area under the graph of the function on the only subset of
Thanks Jo!:-), that’s generous of you. I’ll go through integral cal again :)
Hi,
if possible can you please discuss something about quantum
spaces from categorical approach.
In his lecture on “how to teach differential equations” Rota writes: “Morse theory is a chapter of topology which grew out of Sturm-Lioville theory.” Can someone expand this?
This might be a strange request but here it goes: Am I the only one wishing there were special symbols for “open” resp. “closed subset”? Suppose # was the “open subset” symbol, then one would simply write “thus U # X” or “Let U # X” instead of “thus U is an open subset of X” or “Let U < X be an open subset". I guess I am a bit lazy but if I had a cent for every time a mathematician has to specify that a subset is in fact an open (resp. closed) subset… Maybe a blog post to see how people would feel about introducing such symbols (and finding someone who knows how to create such latex symbols)? I was thinking of something in line with this (one could also do separate for proper (open/closed) subsets): http://img534.imageshack.us/img534/3617/subsets.png
Could you discuss the question how to connect pure maths (maybe especially algebraic geometry) more to the sciences?
There are some obvious (physics) and some less obvious (machine learning, computer science) connections but most people don’t know much about these and I guess there are actually a lot more. And if there are no other connections, it would be very interesting to discuss how to make new connections. This would give pure mathematicians the feeling to do something immediately useful, not only in the far future. At the same time it might be useful to get funding.
One approach to discuss this is found here:
http://a.freshbrain.com/solvr/d/howto-connect-pure-math-sciences
but I’d prefer a distinguished blog like yours for the discussion.
Maybe some of you know something about the current status of “applied algebraic geometry” and the comments will bring to attention the less obvious “applications”.
If you know some other place on the WWW for this question, let me know. In general, I’ve asked this meta-question here:
http://meta.mathoverflow.net/discussion/276/whats-the-right-place-for-opinion-questions/
Konrad, you might be interested in my old post (which might get redone better in the next year or so) on a connection with biology.
A post on the Torelli problem and its current status would be nice.
A review on popular math books. Including the recent “Logicomix”.
I don’t know how Hodge theoretic the people here are. Which version of the Torelli problem are you thinking about? I’m meandering in that direction over at rigtriv (about to start through Andreotti’s proof for Jacobians of Curves)
@#163. I first heard of the Torelli problem together with the question when is a given Abelian variety the Jacobian of a curve. I suppose what I want is an overview taking off right after the construction of Jacobian of a Riemann surface, but more detailed than what is available at Springer Online:
http://eom.springer.de/T/t093260.htm
GS, that question is the Schottky problem, not the Torelli problem. A Torelli theorem is one that says roughly that you can recover a variety from its Hodge theory. For a quick reference, if you can get your hands on LNM 1337, Donagi wrote a survey of the Schottky problem that’s pretty understandable. For Torelli for curves and their Jacobians (the statement being that the map taking a curve to its Jacobian is injective) I’m going to post in that direction starting this week, and talk a bit about things like the Riemann Singularity Theorem and Theta divisors before doing Andreotti’s proof.
Oops, it seems I have completely gone off the rocks. Yes, it is the Schottky problem that I was having in mind. Sorry for the complete screw-up.
But Torelli also will be nice, now that I actually read the Springer online link more carefully…
I’m heading in that direction over at Rigorous Trivialities, though if you’re in more of a hurry, take a look at the Donagi survey I mentioned, and for Torelli, the best proof I’ve seen (Andreotti’s) is written up in Griffiths and Harris.
It appears that V.I. Arnold has died today, 3 june 2010, a sad news indeed. RIP.
Source: http://www.les-mathematiques.net/phorum/read.php?2,602224,602224#msg-602224
I find that at Terry Tao’s blog one can vote up/down comments according as how one likes it. If that were enabled here ….
It might be of interest here to update at times, as well as comment on, what is or isn’t available online from journals and such. The problematic issue is what sources are restricted to subscribers only (often through university libraries). This is an evolving landscape to deal with and gains in importance as a refereed follow-up to informal arXiv preprints or stuff posted on homepages. In e-math today the AMS is announcing free online access to all their journal archives (now scanned):
http://e-math.ams.org/home/page
Just wanted to bring to your attention the plans to shut down the Erwin Schroedinger Institute in Austria:
http://prof-yura.livejournal.com/487154.html
News of the death of Peter Hilton (6 Nov.), in case anyone here wants to comment on his life or work.
http://www.legacy.com/obituaries/pressconnects/obituary.aspx?n=peter-hilton&pid=146500873
This is not a topic, but a request none-the-less. Can you add functionality so that each blog post can be downloaded as a pdf? I would like to read some posts fairly seriously, and this is much more convenient if they are in a pdf(available on several devices, can be used offline, and more ascetically pleasing personally). I expect that this should not be difficult to implement, but I could be wrong.
I’m requesting some blogs on Knutson-Tao honeycombs. They solve a question about Hermitan matrices, but there’s a tropical version as well that seems to have been useful.
This is basically a request for the help of bloggers or others who are in touch with people currently using MathJobs to apply for postdoc positions; there is significant confusion about two openings listed by UMass and the Five Colleges (including UMass). The UMass Dept. of Mathematics & Statistics hopes to hire some recent Ph.D.’s as in the past under multi-year appointments labelled *Visiting Assistant Professor* (VAP). As usual decisions are likely to be very late, but such people are always needed to fill gaps in undergraduate teaching and enhance research life. The Five Colleges have also advertised a POSTDOC in *statistics* only. We’re told that some math applicants are mistakenly checking that box and may get overlooked for math VAP positions. Contact active UMass faculty for further information.
My name is Samuel Hansen and I am responsible for the math podcasts Combinations and Permutations and Strongly Connected Components over at ACMEScience.com and am the co-host of the Math/Maths podcast. I recently started a Kickstarter called Relatively Prime: Stories from the Mathematical Domain. From the project description: “Relatively Prime will be an 8 episode audio podcast featuring stories from the world of mathematics. Tackling questions like: is it true that you are only 7 seven handshakes from the President, what exactly is a micromort, and how did 39 people commenting on a blog manage to prove a deep theorem. Relatively Prime will feature interviews with leaders of mathematics, as well as the unsung foot soldiers that push the mathematical machine forward. With each episode structured around topics such as: The Shape of Things, Risk, and Calculus Wars, Relatively Prime will illuminate each area by delving into the history, applications, and people that underlie the subject that is the foundation of all science.”
I was hoping that the Secret Blogging Seminar could help me get the word out about the project as I think that is is right up your alley.
For more information head on over to http://www.kickstarter.com/projects/386612592/relatively-prime-stories-from-the-mathematical-dom
Hi,
I was wondering if you were willing to start a new blog post discussing the recent opinion piece by Garfunkel & Mumford in the NYT http://www.nytimes.com/2011/08/25/opinion/how-to-fix-our-math-education.html?_r=2
That’s worth discussing in the math bloggosphere I would think (especially if Gowers’ latest blog post is about what I think it is about…)
We know that MO provides public database dumps. There are many important maths blogs on the wordpress software. What in terms of data backups does wordpress allow? Is sbseminar archived in usable form elsewhere?
@Peter, WordPress provides a downloadable xml backup. You can see the latest dump at http://tqft.net/sbseminar/
Hi
I have a question
what is the symplectic leaves of sphere of dimension n when n>2
I don’t know, but it would be useful if you said what Poisson form you are using.
Any explanation on the tiling of Riemann surfaces with connection to an equivalence relation on Poincare disk.
As a monologuist who flunked calculus at Princeton, might I immodestly (and self-servingly) draw your attention to the new DVD of “The Mathematics of Change,” a one-man show I performed at the Mathematical Sciences Research Institute? By the way, the website — http://themathematicsofchange.com — has videos of interviews I did with two MSRI mathematicians, on the burning questions of (a) why 0.999… equals 1 and (b) why zero to the zero power is “undefined.” … Rock on, guys!
Can I suggest taking a look at this recent debate from the UK on the philosophy of mathematics – http://www.iai.tv/video/pythagoras-dream
It features a physicist (Lee Smolin) and a couple of philosophers (Hilary Lawson & Peter Hacker) discussing whether or not mathematics is the underlying structure of reality. Worth a watch at least.
Concerning the Elsevier boycott and related matters, it may be worthwhile to raise the issue of unsolicited “registration” of potential reviewers by journals. I just received an apparently authentic email from the European J. of Combinatorics (Elsevier) stating that I was now registered with them via Elsevier. On the contrary, I have repeatedly told other Elsevier journal editors that I am unwilling to work for their corporation. (One manager wrote back angrily that I was trying to destroy the particular journal.) These automated mailing systems have no opt-out provision, though they do provide a password if you want to visit your “account” with them. To me it all seems unethical. But how to stop it?
P.S. A minor procedural suggestion: if these requests are of any value, it would help to post the most recent ones first.
Hi, all is going sound here and ofcourse every one is sharing facts,
that’s genuinely fine, keep up writing.
Undefeated… But I Can ONLY Scramble…